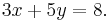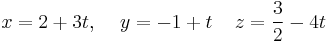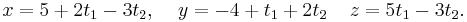Flat (geometry)
In geometry, a flat is a subset of n-dimensional space that is congruent to a Euclidean space of lower dimension. The flats in two-dimensional space are points and lines, and the flats in three-dimensional space are points, lines, and planes. In n-dimensional space, there are flats of every dimension from 0 to n – 1.[1] Flats of dimension n – 1 are called hyperplanes.
Flats are similar to Euclidean subspaces, except that they need not pass through the origin. If Euclidean space is considered as an affine space, the flats are precisely the affine subspaces. Flats are important in linear algebra, where they provide a geometric realization of the solution set for a system of linear equations.
Contents |
Description
A flat can be described by a system of linear equations. For example, a line in two-dimensional space can be described by a single linear equation involving x and y:
In three-dimensional space, a single linear equation involving x, y, and z defines a plane, while a pair of linear equations can be used to describe a line. In general, a linear equation in n variables describes a hyperplane, and a system of linear equations describes the intersection of those hyperplanes. Assuming the equations are consistent and linearly independent, a system of k equations describes a flat of dimension n – k.
A flat can also be described by a system of linear parametric equations. A line can be described by equations involving one parameter:
while the description of a plane would require two parameters:
In general, a parameterization of a flat of dimension k would require parameters t1, ..., tk.
Systematic coordinates for flats in any dimension build on either joins or meets, leading to Grassmann coordinates or dual Grassmann coordinates. For example, a line in three-dimensional space is determined by two distinct points or by two distinct planes. (If the planes are parallel, the ambient space must be a projective space to accommodate a line "at infinity".)
A flat is also called a linear manifold or linear variety.
See also
Notes
- ^ In addition, all of n-dimensional space is sometimes considered an n-dimensional flat as a subset of itself.
References
- Heinrich Guggenheimer (1977) Applicable Geometry,page 7, Krieger, New York.
- Stolfi, Jorge (1991), Oriented Projective Geometry, Academic Press, ISBN 978-0-12-672025-9
From original Stanford Ph.D. dissertation, Primitives for Computational Geometry, available as DEC SRC Research Report 36. - PlanetMath: linear manifold
External links
- Weisstein, Eric W., "Hyperplane" from MathWorld.
- Weisstein, Eric W., "Flat" from MathWorld.